4833: 糖果游戏

Karlsson has visited Lillebror again. They found a box of chocolates and a big whipped cream cake at Lillebror's place. Karlsson immediately suggested to divide the sweets fairly between Lillebror and himself. Specifically, to play together a game he has just invented with the chocolates. The winner will get the cake as a reward.
The box of chocolates has the form of a hexagon. It contains 19 cells for the chocolates, some of which contain a chocolate. The players move in turns. During one move it is allowed to eat one or several chocolates that lay in the neighboring cells on one line, parallel to one of the box's sides. The picture below shows the examples of allowed moves and of an unacceptable one. The player who cannot make a move loses.

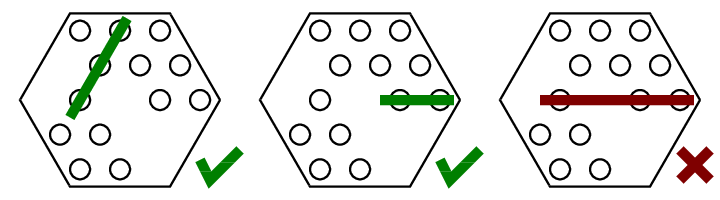
 Karlsson makes the first move as he is Lillebror's guest and not vice versa. The players play optimally. Determine who will get the cake.

Input

The input data contains 5 lines, containing 19 words consisting of one symbol. The word "O" means that the cell contains a chocolate and a "." stands for an empty cell. It is guaranteed that the box contains at least one chocolate. See the examples for better understanding.

Output

If Karlsson gets the cake, print "Karlsson" (without the quotes), otherwise print "Lillebror" (yet again without the quotes).

卡尔松再次造访利勒布罗。他们在利勒布罗家发现了一盒巧克力和一个大鲜奶油蛋糕。卡尔松立即建议他和利勒布罗平分这些糖果。具体来说，就是一起玩他刚用巧克力发明的游戏。获胜者将得到蛋糕作为奖励。

这盒巧克力呈六边形。它包含19个用于巧克力的细胞，其中一些细胞包含一块巧克力。队员们轮流行动。在一次移动过程中，它可以吃一颗或几颗巧克力，这些巧克力位于与盒子一侧平行的一条线上的相邻格子中。下面的图片展示了允许移动和不可接受移动的例子。不能走一步的棋手输了。
卡尔松首先采取行动，因为他是利勒布罗的客人，而不是利勒布罗的客人。玩家发挥最佳状态。决定谁将得到蛋糕。

输入

输入数据包含 5 行，包含 19 个单词，由一个符号组成。单词“O”表示单元格包含巧克力，“.”表示空单元格。保证盒子里至少包含一块巧克力。请参阅示例以更好地理解。

输出

如果Karlsson得到蛋糕，打印“Karlsson”（不带引号），否则打印“Lillebror”（再次不带引号）。

Examples

Input

  . . .
 . . O .
. . O O .
 . . . .
 . . .

Output

Lillebror

Input

  . . .
 . . . O
. . . O .
 O . O .
 . O .

Output

Karlsson